Canonical submersion theorem

Let $f : M \to N$ be a submersion at $p \in M$ , then $m = \dim M \geq n = \dim N$ and there exist charts $(φ_{1}, U_{1}, V_{1})$ around $p$ and $(ψ_{1}, X_{1}, Y_{1})$ around $f (p)$ such that

ψ_{1} \circ f \circ φ_{1}^{- 1} = {π |}_{V_{1}} .

Canonical submersion theorem

REMARK: As can be deduced from the following proof, a submersion gives to $M$ the structure of a product around $p$ since we can construct a diffeomorphism

t_{p} : p \in U_{2} ⟶ f (U_{2}) \times I_{p}^{m - n}

being $I_{p} \subseteq R$ an interval depending on $p$ .

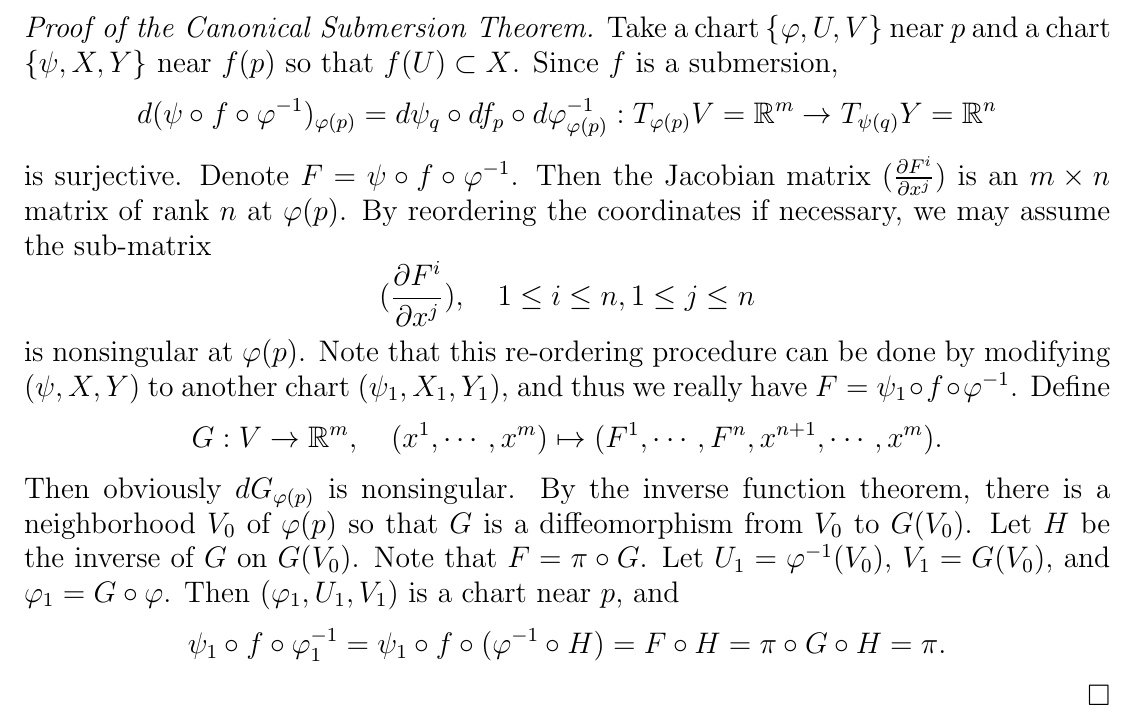
In some sense this is "dual" to the canonical immersion theorem.

The proof use the inverse function theorem.