Poincare half plane

It is a model for the axiomatic hyperbolic geometry.
It consists of the upper half complex plane

H = {x + y i : y > 0}

together with the metric

d \hat{s} = \frac{d s}{y},

or, in other words,

g = (\begin{matrix} \frac{1}{y^{2}} & 0 \\ 0 & \frac{1}{y^{2}} \end{matrix}) .

A subgroup of the Moebius transformations of the Riemann sphere acts on $H$ .

There is a conformal map from the pseudosphere to $(H, g)$ .

What about their geodesics? Since this is a conformal map (in the usual sense of the word "map") the metric can be seen like this
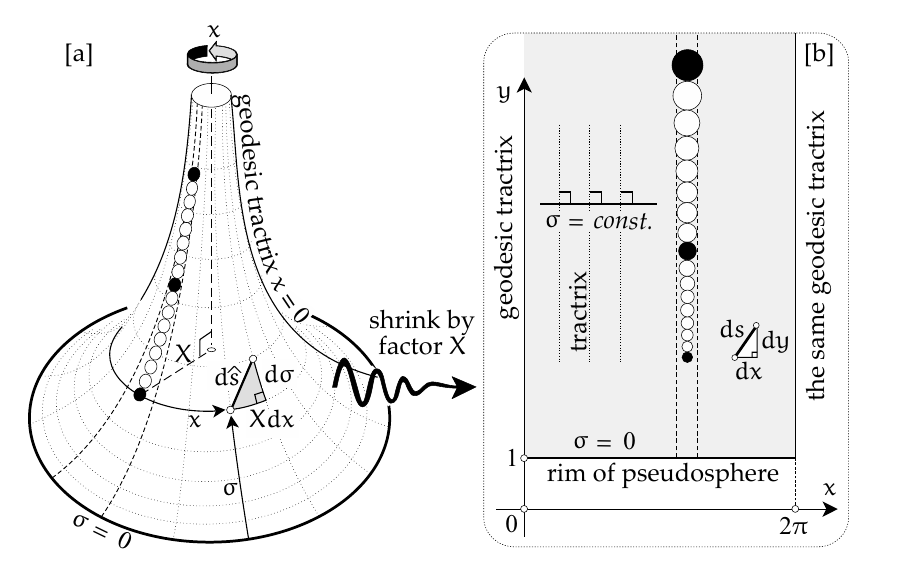
Pasted image 20220105094022.png

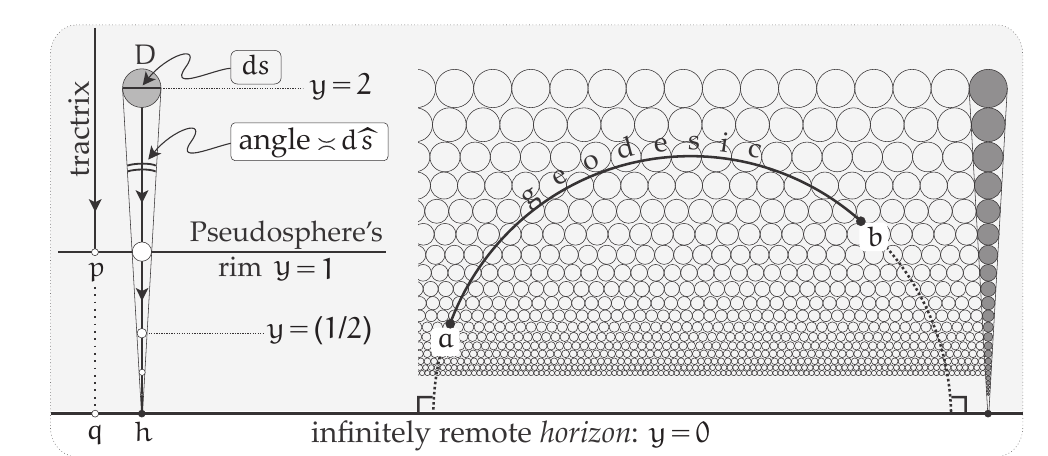
Pasted image 20220105094807.png
See @needham2021visual page 55-57 for details. Every little circle in the map represent a unit of length in the pseudosphere. The conformal requirement is for the circles not to be ellipses. With this picture in mind, geodesics will be lines which go through the fewest amount of circles.
It is related to Snell law for the relation between the angles of light going through two different materials.

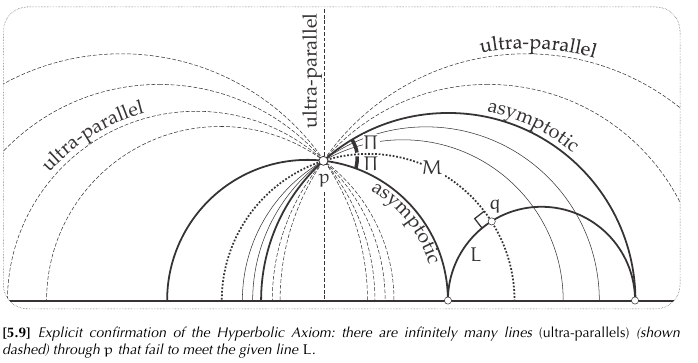
Infinite parallels

See @needham2021visual page 61:
Pasted image 20220614173015.png

Map to the Poincare disk

Define the complex coordinate $z = x + i y \in H$ , and define the new complex coordinate $u$ by the relation $$iu = \frac{1+iz}{1-iz} \qquad \Leftrightarrow \qquad iz = \frac{-1+iu}{1+iu} , . $$ It is easy to check that this establishes a bijection between $H$ parametrized by $z$ and the Poincaré disk $$\mathbb{D} = { u \in \mathbb{C} \mid |u|<1 } , . $$ The metric on the hyperbolic plane and the Poincaré disk in complex coordinates are

g_{H} = \frac{d z d \bar{z}}{(Im z)^{2}} = \frac{4 d u d \bar{u}}{(1 - | u |^{2})^{2}} .

One can check that the Ricci scalar curvature is again constant and equal to $R = - 2$ .

Tridimensional version

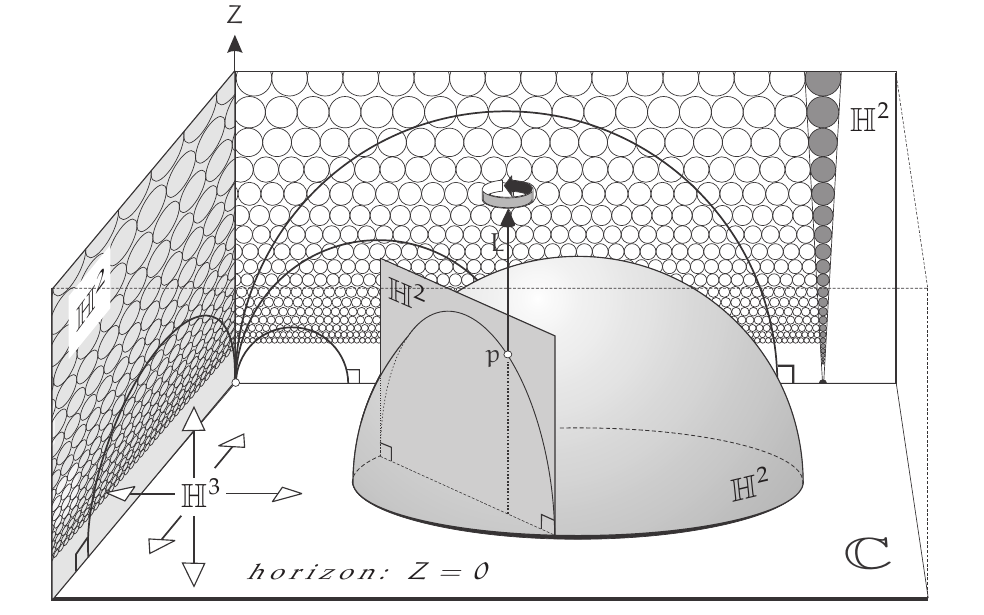
Pasted image 20220116214636.png
See @needham2021visual page 80.