Complexification of a vector space

Sea $V$ un espacio vectorial real, y $J : V \to V$ un $R$ -isomorfismo tal que $J^{2} = - I d$ . Entonces $J$ es llamada una complex structure en $V$ . Cuando en un espacio vectorial real tenemos una tal estructura definida, podemos ver ese conjunto $V$ como un espacio vectorial complejo de la siguiente manera:

(α + i β) \cdot v = α v + β J (v)

Es fácil comprobar que con esta operacion $V$ es un $C$ -espacio vectorial. We will denote it by $V_{J}$ .

Recíprocamente, si $V$ es un espacio vectorial complejo, se puede considerar como un espacio vectorial real restringiendo los escalares. Y ademas, el automorfismo consistente en multiplicar por $i$ es una estructura compleja.

En particular, consideremos $C^{n}$ . Si lo identificamos con el $R$ -espacio vectorial $R^{2 n}$ , la multiplicación por $i$ induce la estructura compleja

J (x_{1}, y_{1}, x_{2}, y_{2}, \dots) = (- y_{1}, x_{1}, - y_{2}, x_{2}, \dots)

cuya matriz en la base canónica es

M = (\begin{array}{ccccc} 0 & - 1 & 0 & 0 & \dots \\ 1 & 0 & 0 & 0 & \dots \\ 0 & 0 & 0 & - 1 & \dots \\ 0 & 0 & 1 & 0 & \dots \\ \dots & \dots & \dots & \dots & \dots \end{array})

con bloques

(\begin{array}{cc} 0 & - 1 \\ 1 & 0 \end{array})

en la diagonal.

El automorfismo $J$ recibe el nombre de estructura compleja estándar, y es única en el siguiente sentido:

Lema

Dada cualquier estructura compleja $J_{1}$ de $R^{2 n}$ , existe una matriz regular $A$ tal que $M_{1} = A \cdot M \cdot A^{- 1}$ , donde estamos denotando por $M$ y $M_{1}$ a las matrices de los morfismos $J$ y $J_{1}$ en la base canónica de $R^{2 n}$ .

Proof

Llamemos $C_{1}^{n}$ al espacio vectorial complejo originado por $R^{2 n}$ junto con la estructura compleja $J_{1}$ , y sea ${f_{1}, f_{2}, \dots}$ una base de dicho $C$ -espacio vectorial. Entonces

{f_{1}, J_{1} (f_{1}), f_{2}, J_{1} (f_{2}), \dots}

es también base de $R^{2 n}$ , con la particularidad de que en dicha base la matriz de $J_{1}$ es

(\begin{array}{ccccc} 0 & - 1 & 0 & 0 & \dots \\ 1 & 0 & 0 & 0 & \dots \\ 0 & 0 & 0 & - 1 & \dots \\ 0 & 0 & 1 & 0 & \dots \\ \dots & \dots & \dots & \dots & \dots \end{array})

Si $A$ es la matriz del cambio de base se tiene que

M_{1} = A \cdot M \cdot A^{- 1} .

$◼$

Así pues, el espacio cociente $\frac{G L (2 n, R)}{G L (n, C)}$ determina todas las estructuras complejas de $R^{2 n}$ mediante la aplicación $[A] \to A \cdot M \cdot A^{- 1}$ .
Para comprobarlo primero necesitaremos el siguiente

Lema

Sea $V$ un espacio vectorial real con una estructura compleja $J$ , y $h$ una aplicación $R$ -lineal de $V$ en si mismo. El endomorfismo $h$ es $C$ -lineal si y sólo si $h (J v) = J h (v)$ .

Proof

Trivial. $◼$

Así pues, consideremos en $\frac{G L (2 n, R)}{G L (n, C)}$ las clases $[A] = [B]$ , entonces las matrices $A M A^{- 1}$ y $B M B^{- 1}$ son iguales, pues $A B^{- 1} \in G L (n, C)$ y por tanto conmuta con $M$ , luego

A M A^{- 1} = A M (A^{- 1} B) (B^{- 1} A) A^{- 1} =

= A (A^{- 1} B) M (B^{- 1} A) A^{- 1} = B M B^{- 1} .

Definition

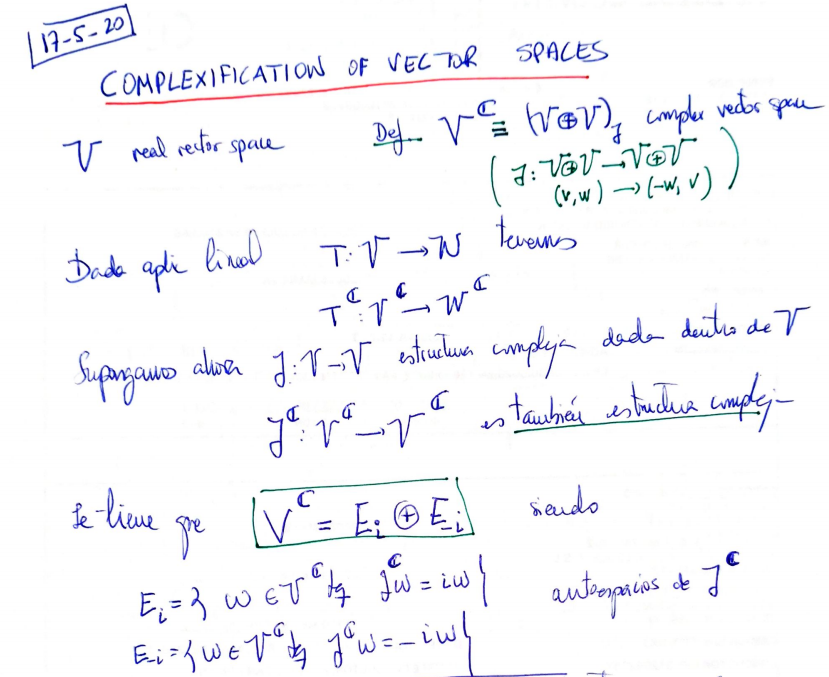
Given a real vector space $V$ , we call the complexification of $V$ , and denote it by $V^{C}$ , to the set $V \oplus V$ with the complex structure given by

J : V \oplus V \rightarrow V \oplus V $$such that $J(v, w)=(-w, v)$. Obviously, $V^{\mathbb{C}}$ can be treated like a complex vector space. This can be constructed, alternatively, using tensor product. See the document in Calibre Complexification, complex structures, inner products, symplectic forms and linear differential equations'' for a good outline of this subject. ------complex eigenvalues--- # Complexification and conjugation Given a vector space $V$ and its complexificated $V^{\mathbb{C}}$, we have a map $C$ (conjugation) that is involutive, $C^2=Id$, and such that the subspace of $V^{\mathbb{C}}$ sucht that

C(v)=v

i s j u s t t h e p r o p e r $ V $ . B u t, g i v e n a n y o t h e r m a p l i k e $ C $, s a y $ D $, t h e s u b s p a c e s u c h t h a t

D(v)=v

is a real vector space, call it $W$, such that $V^{\mathbb{C}}=W^{\mathbb{C}}$. See \begin{verbatim} https://math.stackexchange.com/questions/1061823/conjugation-map-on-a-complex-vector-space \end{verbatim} and TRTR page 414. ![Pasted image 20211230100427.png](/img/user/imagenes/Pasted%20image%2020211230100427.png)