States and observables

From a philosophical point of view:

• States: real world configurations. It is an abstract set, it is probably a primary notion, and cannot be further explained.
• Observable: standardized procedure that let us associate a real number to a state. In the same way, I don't think it can be further explained.
  They both constitute dynamical systems, with a time evolution.

Example: a simplified real world like a bouncing ball in a billiard table has different configurations. We can device different standardized procedures to obtain real numbers: measuring the $x$ position from the lower left corner, measuring the $x$ position from the lower right corner, measuring the $x$ position from another point, or with different rods,...

General scheme, for both Classical Mechanics and Quantum Mechanics:

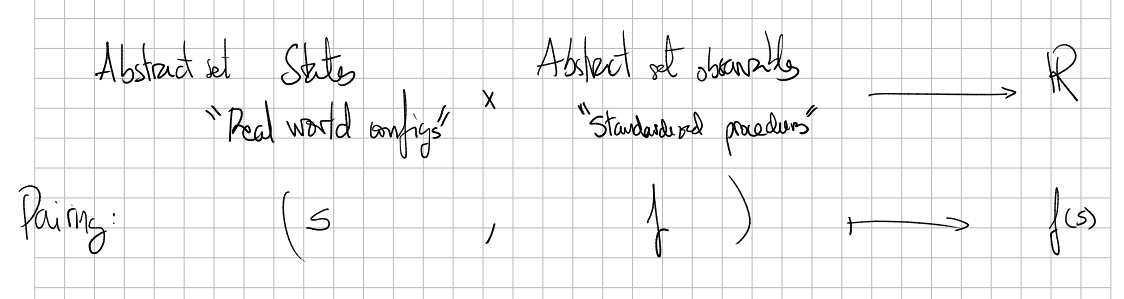
From here it is easy to see Heisenberg vs Schrodinger picture for both, QM and CM:

• if the state changes (the configuration of the world is changing) and the observable is fixed then we obtain different measured values. This is Schrodinger picture
• if the state is considered fixed and the observable changes (I am changing from one standardized procedure to another, for example, I am measuring with my sticks and rods starting in a different initial position, and/or the length of the rods is changing) then we are in Heisenberg picture. See also flow of observables in CM.

(To be thought: Is this the same as general covariance and contravariance? And, what about gauge theory? )

Now we can continue with On Physics from the beginning.