Local Gauss-Bonnet theorem
Let
where
This theorem says that the angular excess of such a triangle is simply the total curvature inside it.
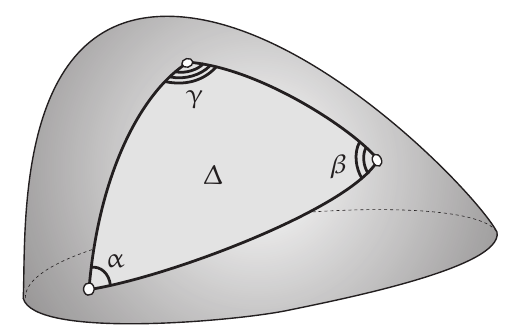
There is a visual idea of the proof in @needham2021visual page 23:

based on the additivity of the angular excess.
It has a surprising global version: global Gauss-Bonnet theorem.